In geometry, cone is a solid or hollow object with a round flat base and sides that slope up to a top point. The cone formulas, solved example & step by step calculations may useful for users to understand how the input values are being used in such calculations. Also this featured cone calculator uses the various conversion functions to find its area, volume & slant height in SI or metric or US customary units. A right circular cone is a cone where the cone's axis is the line meeting the vertex to the mid-point of the circular base. The centre of the circular base is joined with the apex of the cone, and it forms a right angle.
A right circular cone is a cone in which the altitude or height is exactly perpendicular to the radius of the circle. In comparison, a cone is a \(3D\) figure with one curved surface and a circular base. A cone is a solid having a circular base and whose lateral surface tapers into a point. And this line joining the vertex to the circular base is called the axis of the cone. When this axis is perpendicular to the base at the center O of the circular base, we say the cone is a right circular cone.
Let's learn how to calculate the volume of a cone but before that look at the definition of the cone. If the cone is right circular the intersection of a plane with the lateral surface is a conic section. In general, however, the base may be any shape and the apex may lie anywhere . Contrasted with right cones are oblique cones, in which the axis passes through the centre of the base non-perpendicularly. If a cone and cylinder have the same height and base radius, then the volume of cone is equal to one third of that of cylinder. That is, you would need the contents of three cones to fill up this cylinder.
The same relationship holds for the volume of a pyramid and that of a prism . Let's get right to it — we're here to calculate the surface area or volume of a right circular cone. As you might already know, in a right circular cone, the height goes from the cone's vertex through the center of the circular base to form a right angle. Right circular cones are what we typically think of when we think of cones.
With the Pythagorean theorem, use the radius and the height to calculate the slant height of the cone, then multiply the slant height by the radius by pi. To that you add the base area of the cone, which is found by multiplying pi by the square of the radius. The total surface area is found by adding the lateral surface area to the base area. In geometry, a cone is a 3-dimensional shape with a circular base and a curved surface that tapers from the base to the apex or vertex at the top.
In simple words, a cone is a pyramid with a circular base. In a cone, the base radius 'r' is given as 7 cm and the slant height 'l' is given as 25 cm. In geometry, a cone is a solid figure with one circular base and a vertex. The height of a cone is the distance between its base and the vertex.
The cones that we will look at in this section will always have the height perpendicular to the base. Conical and pyramidal shapes are often used, generally in a truncated form, to store grain and other commodities. Similarly a silo in the form of a cylinder, sometimes with a cone on the bottom, is often used as a place of storage. It is important to be able to calculate the volume and surface area of these solids. The "height" of a cone, and the "slant height" of a cone are not the same thing. The height of a cone is considered the vertical height or altitude of the cone.
This is the perpendicular distance from the top of the cone down to the center of the circular base. The slant height of a cone is the distance from the top of the cone, down the side of the cone to the edge of the circular base. Given slant height, height and radius of a cone, we have to calculate the volume and surface area of the cone. Find the curved surface area of a cone with base radius 5.25 cm and slant height 10 cm.
In the field of geometry calculations, finding the area, volume & slanting height of a cone is very important to understand a part of basic mathematics. In this module, we will examine how to find the surface area of a cylinder and develop the formulae for the volume and surface area of a pyramid, a cone and a sphere. These solids differ from prisms in that they do not have uniform cross sections.
A cone is a three dimensional geometric shape with one vertex and a circular base. The line form the centre of the base to the apex is the perpendicular height. If the enclosed points are included in the base, the cone is a solid object; otherwise it is a two-dimensional object in three-dimensional space. The slant height of a cone should not be confused with the height of a cone.
Slant height is the distance from the top of a cone, down the side to the edge of the circular base. Slant height is calculated as \(\sqrt\), where \(r\) represents the radius of the circular base, and \(h\) represents the height, or altitude, of the cone. To calculate the slant height of either a cone or a pyramid, you need to imagine that you can look inside of the figure.
First, we cut down through the cone from vertex point A to segment BC to get two halves. The cut surface of either half is now in the shape of an isosceles triangle, which is a triangle with two sides that are the same length. Those two sides were the slant height of the cone.
We now have triangle ABC, where sides AB and AC have the same length. In our everyday life, we come across different types of cones. You can easily find out the volume of a cone if you have the measurements of its height and radius. In a cone, the perpendicular length between the vertex of a cone and the center of the circular base is known as the height of a cone.
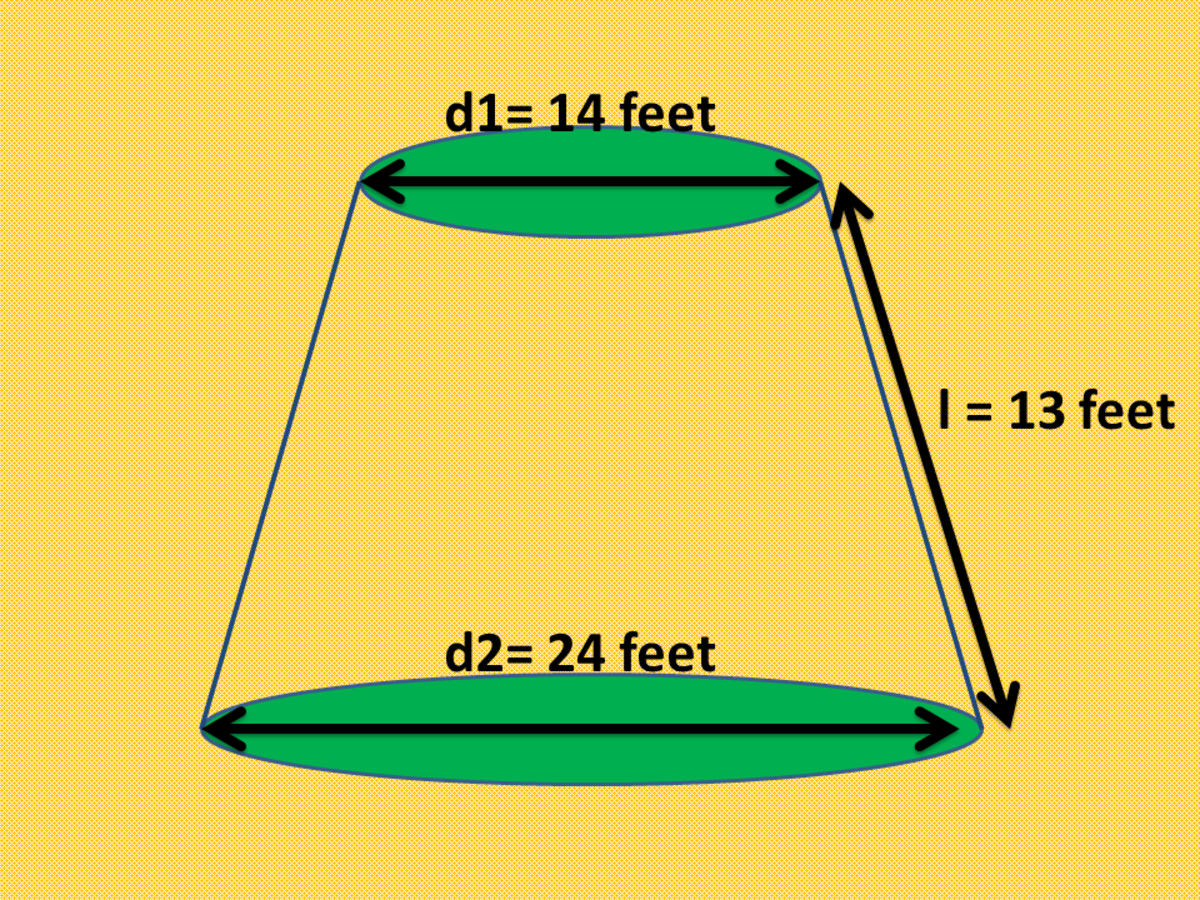
A cone's slanted lines are the length of a cone along the taper curved surface. All of these parameters are mentioned in the figure above. A conical tent is 10 m high and the radius of its base is 24 m. If the cost of 1 m2 canvas is Rs 70, find the cost of the canvas required to make the tent. The slant height and base diameter of a conical tomb are 25 m and 14 m respectively.
Find the cost of white-washing its curved surface at the rate of Rs 210 per 100 m2. Now there are two cones with base radius and slant heights as, and, respectively. Now there are two cones with base radius and slant heights as , & , respectively. Find the area of canvas required for a conical tent of height 24 m and base radius 7 m.
Monica has a piece of canvas whose area is 551 m square. She uses it to have a conical tent made , with a base radius of 7 m. Now let us locate the centre of the base and name it O. From this center we measure distance to any point on the circumference on the top. We have to draw a circle with slant height as radius and mark an arc of x cm.
Draw a sector AOB with L as radius and length of the arc as x cm . Our traffic cone is a little different from the geometric shape called a cone. In geometry, the base of a cone is only a circle that does not extend beyond the opening of the cone. The point of a cone in geometry is called the vertex point.
The slant height and the altitude always meet at that vertex point in a cone. On the traffic cone, the two segments did not meet because the tip is flat and does not come to one point. A cone is a three-dimensional figure with one circular base. A curved surface connects the base and the vertex.
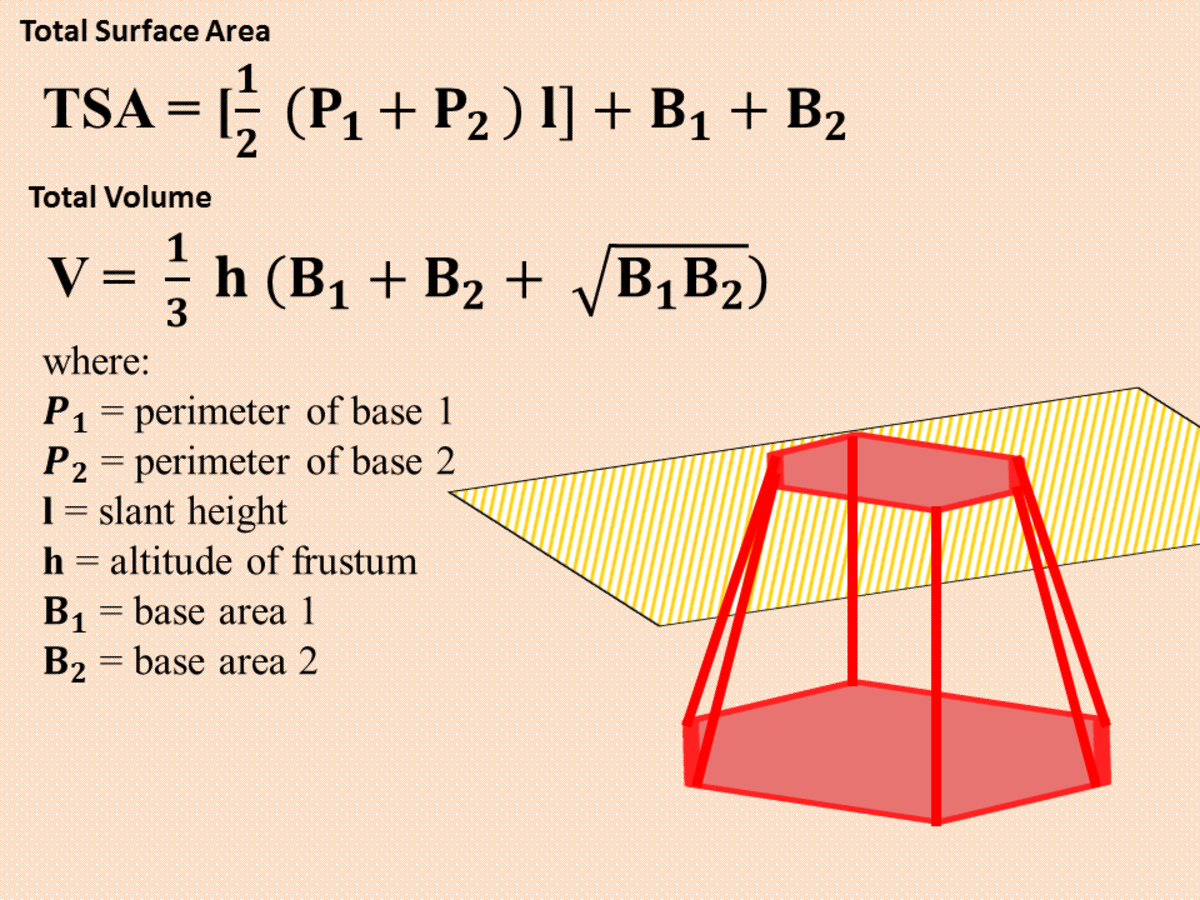
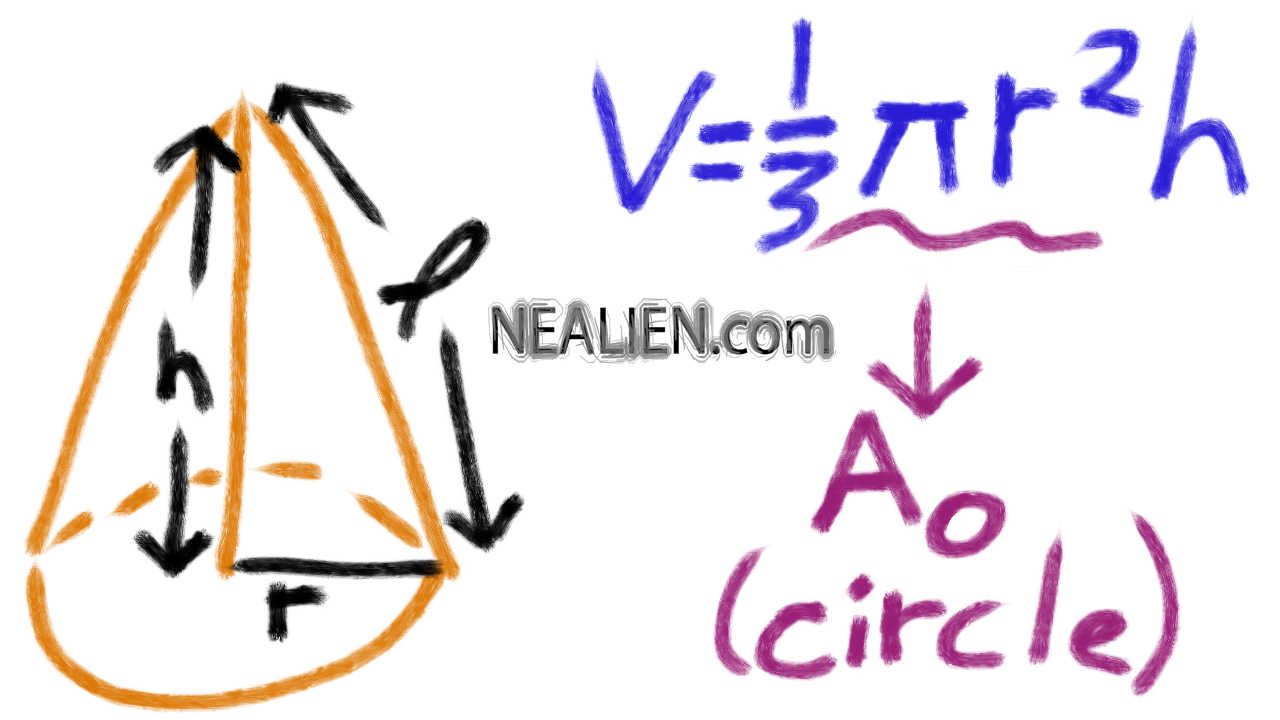
Given height and slant height calculate the radius, volume, lateral surface area and total surface area. Given radius and slant height calculate the height, volume, lateral surface area and total surface area. Given radius and height calculate the slant height, volume, lateral surface area and total surface area. In figure A is called vertex, AO is height, OC is radius, and AC is slant height of cone. If you don't know the height or the radius, you can use the slant height to obtain some of the other data, this using the Pythagorean theorem.
You can also use the online calculator to get the volume of a oblique cone automatically . In case the vertex moves outside the space of the base, it is necessary to calculate the height with respect to the same base, always keeping the angle of 90 degrees. A tent is in the form of a right circular cylinder surmounted by a cone. The height of the cylindrical portion is 11 m while the vertex of the cone is 16 m above the ground. Find the area of the canvas required for the tent.
The radius of the cone is the radius of the base. The altitude of the cone is the perpendicular segment from the vertex to the plane of the base. The height of the cone is the length of the altitude.
The slant height of a right cone is the length of the segment from the vertex of the cone to the circle of the base. Following figure shows the cone with base radius r, slant height l and height h. The cone is the three-dimensional geometrical figure or object which has the base in the form of circle of some radius. This is an ideal calculator for a teacher to make up some interesting questions, as it provides all the parameters of the formula. This right circular cylinder and a right circular cone are of the same base radius and the same height.
In this article, we have learnt the definition of cone and the right circular cone, and we discussed daily-life examples of the right circular cone. We will still observe some vacant space in the container. Repeat this experiment once again; we will notice this time the cylindrical container is completely filled.
Thus, the volume of a cone is equal to one-third of the volume of a cylinder having the same base radius and height. Think of volume as the amount of liquid that you could fill an object with, and think of surface area as how much paper you could wrap over that object. Every cube, sphere, cylinder, cone , and so on has a volume and a surface area; and the formulas used for finding these measurements is different for each shape. For a right cone, the lateral surface area is πrl, where r is the radius and l is the slant height.
A cone has a three-dimensional shape so calculating its volume can seem a little complicated. To help you understand better, in this article we explain what a cone is as well as how to calculate its volume. We detail the steps one by one and the formulas you have to use to calculate the volume of a cone with accurate examples. An oblique cone is a cone with an apex that is not aligned above the center of the base. It "leans" to one side, similarly to the oblique cylinder.
The cone volume formula of the oblique cone is the same as for the right one. We can conclude from this experiment that the volume of a cylinder is thrice the volume of a cone having the same height and base radius. This implies that the volume of a cone is equal to one-third of the volume of the cylinder having the same height and base radius. Find the total surface area of a cone, whose base radius is 3 cm and the perpendicular height is 4 cm.
Given slant height and lateral surface area calculate the radius, height, volume, and total surface area. Given height and volume calculate the radius, slant height, lateral surface area and total surface area. Given radius and total surface area calculate the height, slant height, volume and lateral surface area.
Given radius and lateral surface area calculate the height, slant height, volume and total surface area. Given radius and volume calculate the height, slant height, lateral surface area and total surface area. The oblique cone is the same solid shape as the regular cone, it has two faces, a circle as a base, and a curved face, one vertex and one edge. The difference between the oblique cone and the regular cone is that its vertex is not aligned in the center. In other words, the vertex does not form a 90 degree angle with the center of the base.
The total amount of canvas required would be equal to the curved surface area of the cone. A circus tent is cylindrical to a height of 3 metres and conical above it. If its diameter is 105 m and the slant height of the conical portion is 53 m, calculate the length of the canvas 5 m wide to make the required tent. Now there are two cones with base radius, slant height and Curved Surface Area (C.S.A) as,, , , respectively. The area of the curved surface of a cone is 60πcm2. If the slant height of the cone be 8 cm, find the radius of the base.
Find the curved surface area of a cone, if its slant height is 60 cm and the radius of its base is 21 cm. Now there are two cones with base radius, slant height and Curved Surface Area (C.S.A) as , , & , , respectively. Monica has a piece of Canvas whose area is 551 m2.